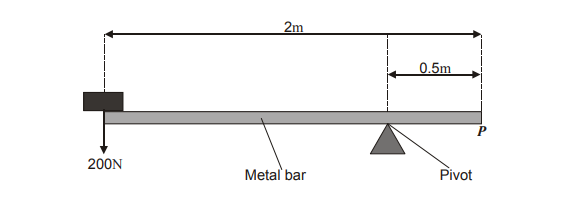
@AriHoresh
For a uniform bar to be “stable” or in equilibrium, the Clockwise Moment must equate the Anti-Clockwise moment around the pivot.
A moment (M) in Newton meters is equal to the product of the Force (F) in Newtons and the perpendicular distance from the pivot (d) in meters.
M=Fd
With the provided values we are able to deduce the Anti-clockwise moment.
However beforehand, we must look at any additional information that may come into play.
Here, this information, or trick per say, is “A uniform bar of length 2.0 m and weight 1000 N has its centre of gravity at its centre”.
This means that for the Anti-clockwise moment, we must calculate 2 sets of values and add them to give us the final Anti-clockwise moment-
So it will look something like this:
M_{A1}= F \times d= 200N \times (2m - 0.5 m) = 200N \times 1.5 = 300 Nm
M_{A2} = F \times d = 1000N \times 0.5m = 500 Nm
Since the weight of the bar acts on it’s center of gravity at the centre of the bar (1m), the distance from the pivot will be 0.5m
M_{AT}= M_{A1} + M_{A2} = 300 Nm + 500 Nm = 800 Nm
Now, we proceed to look at the Clockwise Moment-
M_{C}= F \times d = 0.5F
We learn that we need to find this force or weight, F.
We can use the concept of Anti-clockwise moment= Clockwise Moment to find it:
M_{AT}= M_{C},
where M_{AT}= 800 Nm and M_{C}= 0.5F
So we can say
0.5F= 800 Nm
F= {\frac {800 Nm}{0.5 m}}= 1600 N
Which makes the weight needed at Position P to balance the bar 1600 N or choice (D).