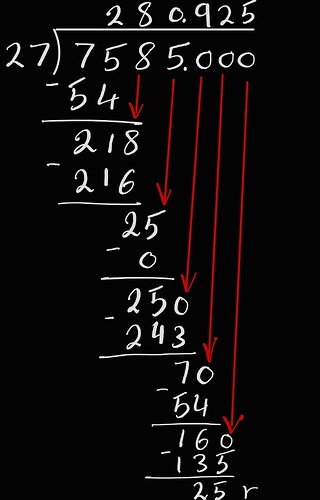
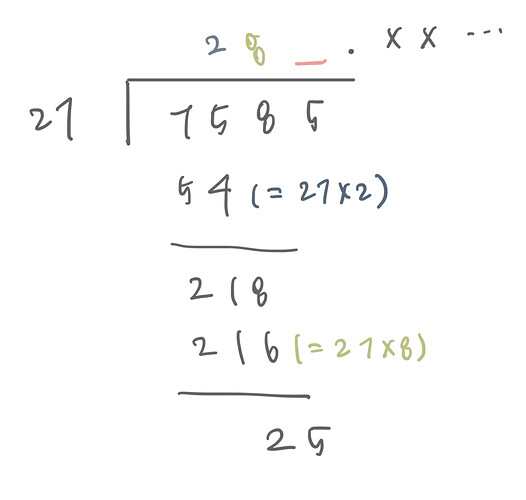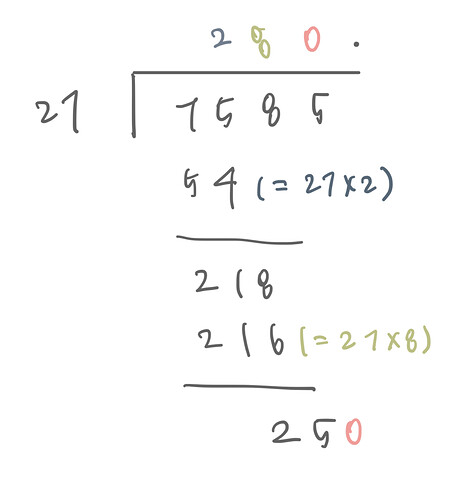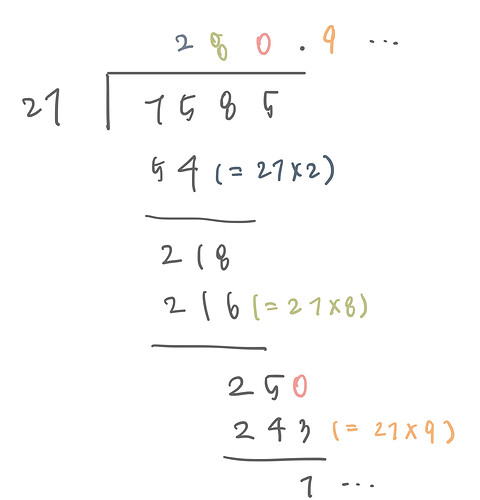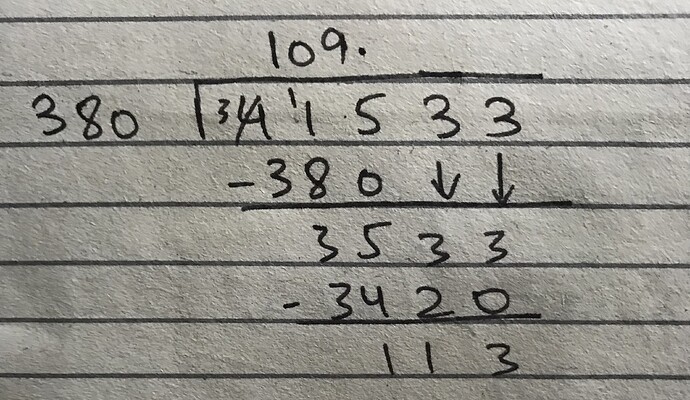Can anyone solve 7585/27?
Thank you 
Can you please tell me how did you put 0 after 28 in the quotient?
I think division is like finding the closest multiplication
to make the remainder as small as possible.
We did 27 * 2 = 54 to get smallest remainder in 75 (21)
and 27 * 8 = 216 to again, get smallest remainder in 218 (2)
Now we want to do the same thing with 25. We want to divide 25 with some number to continue with the division, but 25 is smaller than 27.
This is why we add 0.
Then it’s gonna look like this:
Now since 250 is bigger than 27, we can continue the division like:
Hope this helps 
1 Like
So whenever the remainder is smaller than the divisor, we will solve it by adding a zero in the quotient?
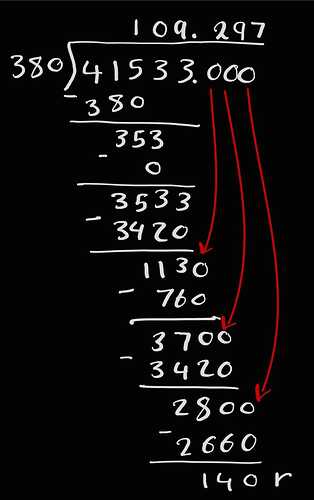
But the same is happening when we are dividing 41533/380.
The complete answer is 109.291 instead of 109.0291 @winter1 please can you clarify this?
Thanks alot. Can you solve 7585/27 please?
Thanks alot. I really like the methodology of adding .000 in the dividend. My question: Is there a specific rule to add 3 zeros after the decimal in the dividend or we can add as many zeros as we want?
however many zeros as you want!
Also last question (hopefully)
In the first question that you solved, the remainder was 113 which was also smaller than the divisor i.e. 380. Then why we didn’t place a zero in the quotient before placing a decimal?
After subtraction, you always have to move one number from the dividend down to the product of subtraction. Why? Because the product of subtraction always has to be smaller than the divisor, if it wasn’t then you’ve made a mistake in the previous step. Now, only after you’ve moved one number from the dividend whether it’s an actual number that was part of the original dividend or just a zero placed after a decimal, down to the subtraction product, can you determine whether or not there’s any equal value to the divisor in the product you’re working on.
In the 113 you refer to, that was the pure product of subtracting 3420 from 3533, only after adding the next number from the dividend to the 113 can I begin to divide it by the divisor and place a number in the quotient.