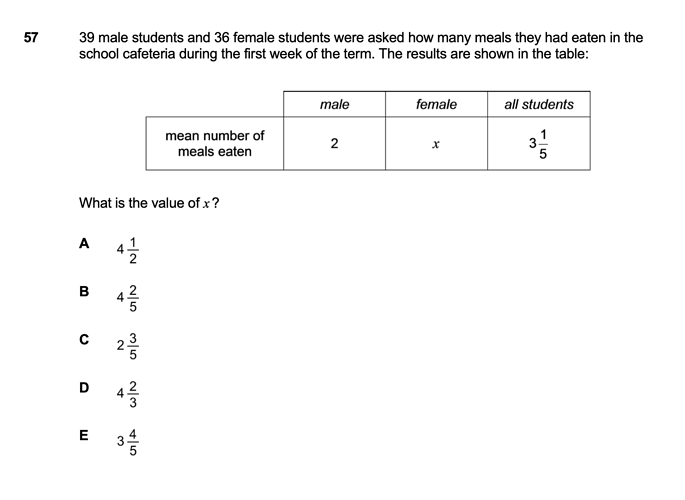
In order to solve this, we must make 3 equations:
39 → number of male students
36 → number of female students
75 → total number of students
M_m= \frac{\text{Sum of all meals eaten by male (using m as a variable)}}{ \text{Number of male students}}
M_f= \frac{\text{Sum of all meals eaten by female (using f as a variable)}}{ \text{Number of female students}}
M_t= \frac{\text{Sum of all meals eaten by male and female}}{ \text{Number of total students}}
So, in our situation:
M_m= \frac{m}{39} = 2 \to m = 39 \cdot 2 \to m = 78.
M_t= \frac{m+f}{75} = 3 \frac{1}{5}= \frac{16}{5} \to m+f = 75 \cdot \frac{16}{5} = 240
\to f = 240-m = 240 - 78 \to f = 162.
Using that information, we can calculate the mean number of meals eaten by female students as:
M_f = \frac{f}{36} = \frac{162}{36} = \frac{81}{18} = \frac{9}{2} = 4\frac{1}{2}.
So the correct answer is (A)
why do we get 16/5? shouldn’t it be 3/5?
as you can see it is 3 full 1/5 which is equal to 16/5