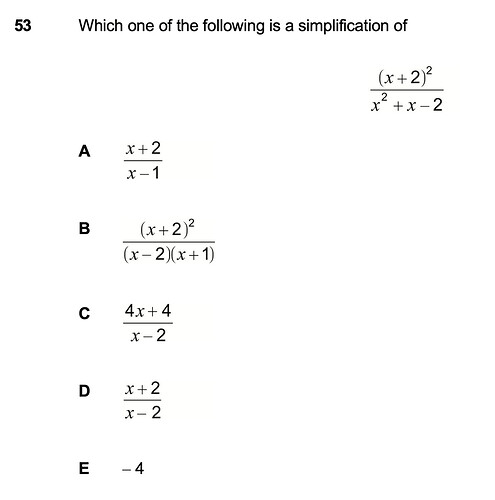
Let’s start simplifying:
\frac{(x+2)^2 }{x^2+x-2} = \frac{(x+2)^2 }{x^2+2x -x-2} = \frac{(x+2)^2 }{x( x+2) -(x+2)}= \frac{(x+2)^2 }{(x-1)( x+2)} = \frac{x+2 }{x-1}.
So the correct answer is (A)
p.s. There is also another way to find the roots of x^2+x-2 and that is using the formula x_{1,2}= \frac{-b \frac{+}{} \sqrt{b^2 -4ac}}{2a} where the equation is in the form ax^2 +bx +c. Using the roots you get ax^2 +bx +c = (x-x_1)(x-x_2).
6 Likes