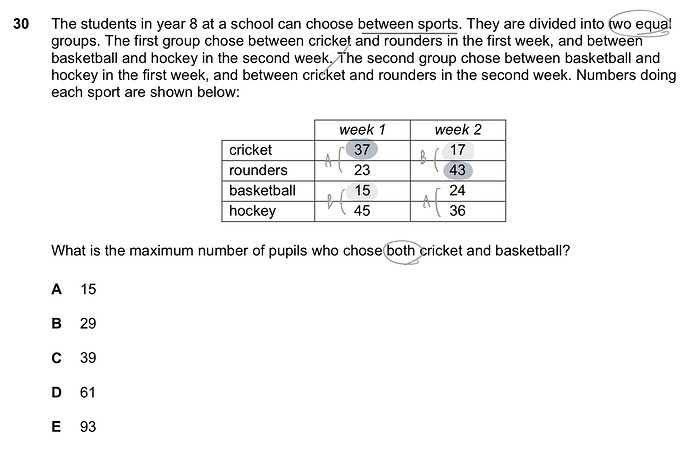
Hi, can I please get any help on how I should approach these types of questions?
is the answer c. 39?
Yes, just checked and it’s true. ![]()
Well, the way I did it is kinda like this:
The keyword in the question is maximum number. This actually is very important, not only does it make the question relatively easy and simple to solve, but also it would be utterly impossible to solve it without it.
So basically, in the 1st group in the first week, 37 students chose cricket. And then in week 2, from the very same group, 24 students chose basketball. To satisfy our hypothetical scenario of finding the maximum number of students who chose both cricket and basketball, we will go ahead and assume that all 24 students who chose basketball in the second week were from the 37 that chose cricket the first week. This is only possible because the number of students who chose basketball (in the 2nd week) is smaller than the number of those who chose cricket. And note here that if it weren’t for the word maximum we wouldn’t have been able to assume that 100% of the 24 students came from the 37. Because for all we know, some of them, if not all, might’ve been from the group that chose rounders.
Now, the second group. Let’s apply the same method. 15 students chose basketball the first time, so we’ll just assume that all 15 who chose cricket in the second week were the same students. But wait! 17 students chose cricket in the 2nd week, what does that mean? well that simply means that 2 students had chosen hockey the first time around, and then chose cricket joining the 15 students who had chosen basketball.
Giving us a grand total of 24 + 15 students.
Edit:
Obviously, I’ve assumed that you know where each group is located on the diagram because you’ve marked the diagram with the correct labels.