Hi,
how do we find 7/13?
That’s odd. I think it should be a fifty fifty probability, meaning 1/2 .
you’ve arrived at the conclusion that the probability of the first boy winning is 1/36. Sure, that would be correct had he been playing by himself, but, he isn’t. You’ve actually only found the probability of getting a combination of 12 which is possible if both dice landed on 6 and therefore the probability would be 1/6 × 1/6 = 1/36 .
Great, but, for the first boy to win he’ll need to get his bet first. In other words, the real question is: In whose favor are the odds? The boy with the more probable bet should win, because obviously, the higher the probability the more likely the event is going to take place first.
So what about the other boy, what are his odds, what is the probability of his bet?
The second boy bet that the combination 7 will come up twice in a row ! Ok, so how do we calculate that?
Well, first, the probability of getting a combination of two numbers that add up to 7 is 6/36 because you have 6 pairs of number that can come up and their sum would equal to 7, this is better explained in this chart:

The formula goes: probability = number of favorable outcomes/overall possibilities
as I’ve explained in the chart, there are 6 favorable outcomes, and obviously the overall possibilities are 36. Therefore: the probability of getting the sum of 7 when throwing two dice is: 6/36 = 1/6
Now the confusing part is that the second boy bet that a combination of 7 will come up twice in row. For that we will use the multiplication rule of two independent events. The events are indeed independent because the outcome of the first throw has no effect whatsoever on the next.
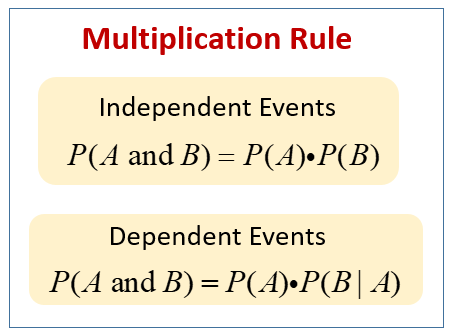
Finally, the probability of getting the combination of 7 twice in a row = the probability of the first event × the second.
the probability of getting the sum of 7 is 1/6
→ 1/6 × 1/6 = wait for it, yup 1/36
The odds of either boy winning are exactly the same.
But that is, of course, all wrong because 7/13 is not 1/2. Though it is very close, only about 0.03 off, and I wonder if that means the method I used was correct to an extent. If you found any explanation do bring me up to date please.
